About Me
Welcome! I am an economist interested in the intersection of microeconomic theory, econometrics, and experimental methods. My research focuses on two related questions: how to uncover the empirical foundations of economic modeling from individual choice data, and how strategic interactions unfold in settings with private information — including decentralized protocols. I am currently a Senior Associate at Charles River Associates in the Antitrust and Competition Practice. Prior to this, I was a Postdoctoral Scholar at the UC Berkeley Department of Economics, where I also earned my Ph.D.You can find Python implementations of different tools that I have developed for my research in my GitHub page.
Please feel free to reach out to me at cristian (at) cristianugarte.com
Research Papers
Preference Recoverability from Inconsistent Choices
We study the analysis of choices imperfectly aligned with the preference relation that drives them. First, we develop a measure of decision-making quality that, unlike the existing ones, ensures to asymptotically measure the distance between the subject's choices and her underlying preference (instead of some preference). We then use such a measure to propose a statistically consistent preference estimator. Empirical results suggest consistency is a relevant property when recovering preferences, especially for complex choice environments, compared to estimators based on intuitive motivations.
The Behavioral Restrictions of a Differentiable Utility
Economic models usually endow agents with (well-behaved) differentiable utilities. However, there is no clarity on what, if any, behaviors are ruled out by making this assumption. I study conditions under which consumer choices can be rationalized by a differentiable utility, i.e., smoothly rationalized. Starting from the observation that differentiability implies that indifferent choices have the same marginal rate of substitution, I develop an exact test for smooth rationalization. I also show that the existence of higher-order derivatives, commonly used for comparative statics, does not impose any behavioral restrictions. I test smooth rationalization into several experimental data sets and find that, in most cases, choices are consistent with a differentiable utility.
The generality of the Strong Axiom
Economic Theory, (2025)
Economic research usually endows consumers with a strictly concave utility function. When choices are rationalizable, this assumption can be tested by the Strong Axiom of Revealed Preferences, SARP, as if they fail such a test, the convexity of the utility is not strict. We extend this test to non-rationalizable choices using partial efficiency, the most popular method to recover preferences. Under partial efficiency, a strictly convex utility cannot be tested. Hence, the existence of a strictly concave utility is falsified if, and only if, choices are rationalizable but fail SARP, which we do not observe in laboratory data. From an empirical standpoint, our results suggest that assuming a strictly concave utility does not carry a cost.
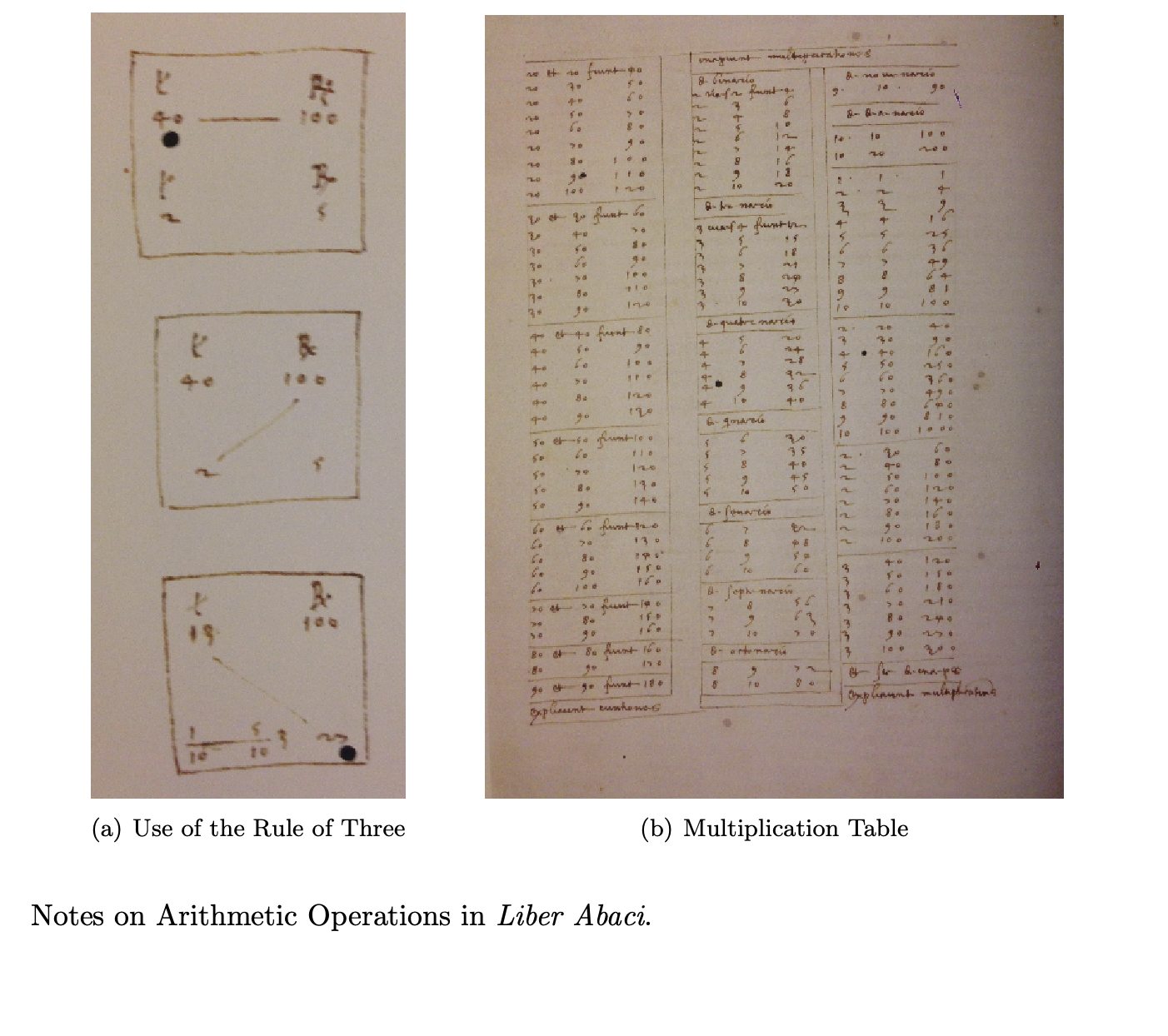
Mathematical Finance, Information Flow, and Economic Growth in pre-industrial Europe
Old (and dormant) work for the Econ History class at UC Berkeley. Not as polished, but still a pretty cool result.
This paper studies the role of financial techniques development in Europe's economic growth from the thirteenth to the seventeenth century. During this era, mathematicians developed the main advances in finance, and Fibonacci’s book Liber Abaci is undoubtedly the most important development. This paper uses the publications of mathematics books as a measure of exposure to new financial techniques, and exploits city-level data. Results suggest that the of exposure to new financial technologies had a causal effect in economic growth before the sixteenth century. The presence of reverse causality and measurement error after the invention of the printing press make the effect impossible to identify after the sixteenth century.